Delving into the intricacies of mathematical operations, roots play a central role in unraveling the essence of numbers and their relationships. Root operations, such as square root, cube root, and fourth root, are pivotal in various mathematical contexts, offering a systematic way to extract roots and solve a myriad of problems.
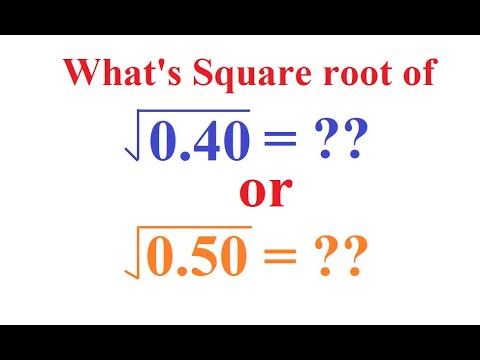
The square root, symbolized by the radical sign (√), is a foundational root operation. It involves finding a number that, when multiplied by itself, equals the given number. This operation is denoted as √x, where x is a non-negative real number. For instance, the square root of 16 is 4, as 4 multiplied by itself results in 16.
Extending the concept, the cube root introduces a new dimension to root operations. Represented by the radical symbol with a small 3 (∛), the cube root seeks a number that, when multiplied by itself twice, equals the original number. As an example, the <a href=”https://rootcalculation.com/cube-root-of-27/”>cube root of 27</a> is 3, as 3 multiplied by itself twice (3 x 3 x 3) equals 27.
Further expanding our exploration, we encounter the fourth root operation, marked by the radical symbol with a small 4 (∜). The fourth root involves finding a number that, when raised to the power of 4, equals the given number. While less commonly encountered than the square and cube roots, the fourth root is a valuable tool in mathematical analysis. For example, the <a href=”https://rootcalculation.com/fourth-root-of-27/“>fourth root of 81</a> is 3, as 3 raised to the power of 4 equals 81.
Understanding these root operations provides a foundation for navigating mathematical landscapes. It is essential to note that roots are not confined to positive real numbers; they can also be extended to include complex numbers and negative real numbers. In the realm of negative real numbers, even-root operations (such as square root and fourth root) yield complex results, while odd-root operations (like cube root) produce real roots.
The applications of root operations extend across diverse fields, from physics and engineering to computer science and finance. In physics, roots are crucial for solving equations related to motion, energy, and wave phenomena. Engineers frequently employ root operations in calculations involving electrical circuits, signal processing, and structural analysis.
In conclusion, root operations stand as indispensable tools in the mathematician’s arsenal, providing a structured approach to unraveling the roots of numbers and solving a broad spectrum of mathematical challenges. From the ubiquitous square root to the less commonly explored fourth root, these operations offer a versatile set of tools for understanding the intrinsic nature of numbers and their intricate relationships. As we journey through mathematical concepts and their applications, the significance of root operations becomes increasingly apparent.













Leave a Reply